Alexander Graham Bell

(Reprint from National Geographic Magazine Vol. XIV, No.6, June 1903)

In 1899, at the April meeting, I made a communication to the Academy upon the subject of " Kites with Radial Wings" and some of the illustrations shown to the Academy at that time were afterwards published in the Monthly Weather Review.
Since then I have been continuously at work upon experiments relating to kites. Why, I do not know, excepting perhaps because of the intimate connection of the subject with the flying-machine problem. We are all of us interested in aerial locomotion; and I am sure that no one who has observed with attention the flight of birds can doubt for one moment the possibility of aerial flight by bodies specifically heavier than the air.
In the words of an old writer, "We cannot consider as impossible that which has already been accomplished."
I have had the feeling that a properly constructed flying-machine should be capable of being flown as a kite; and, conversely, that a properly constructed kite should be capable of use as a flying-machine when driven by its own propellers. I am not so sure, however, of the truth of the former proposition as I am of the latter.
Given a kite, so shaped as to be suitable for the body of a flying-machine, and so efficient that it will fly well in a good breeze (say 20 miles an hour) when loaded with a weight equivalent to that of a man and engine; then it seems to me that this same kite, provided with an actual engine and man in place of the load, and driven by its own propellers at the rate of 20 miles an hour, should be sustained in calm air as a flying-machine. So far as the pressure of the air is concerned, it is surely immaterial whether the air moves against the kite, or the kite against the air.
Of course in other respects the two cases are not identical. A kite sustained by a 20-mile breeze possesses no momentum, or rather its momentum is equal to zero, because it is stationary in the air and has no motion proper of its own; but the momentum of a heavy body propelled at 20 miles an hour through still air is very considerable. Momentum certainly aids flight, and it may even be a source of support against gravity quite independently of the pressure of the air. It is perfectly possible, therefore, that an apparatus may prove to be efficient as a flying-machine which cannot be flown as a kite on account of the absence of vis viva.
However this may be, the applicability of kite experiments to the flying-machine problem has for a long time past been the guiding thought in my researches.
I have not cared to ascertain how high a kite may be flown or to make one fly at any very great altitude. The point I have had specially in mind is this: That the equilibrium of the structure in the air should be perfect; that the kite should fly steadily, and not move about from side to side or dive suddenly when struck by a squall, and that when released it should drop slowly and gently to the ground without material oscillation. I have also considered it important that the framework should possess great strength with little weight.
I believe that in the form of structure now attained the properties of strength, lightness, and steady flight have been united in a remarkable degree.
In my younger days the word "kite" suggested a structure of wood in the form of a cross covered with paper forming a diamond-shaped surface longer one way than the other, and provided with a long tail composed of a string with numerous pieces of paper tied at intervals upon it. Such a kite is simply a toy. In Europe and America, where kites of this type prevailed, kite-flying was pursued only as an amusement for children, and the improvement of the form of structure was hardly considered a suitable subject of thought for a scientific man.
In Asia kite-flying has been for centuries the amusement of adults, and the Chinese, Japanese, and Malays have developed tailless kites very much superior to any form of kite known to us until quite recently.
It is only within the last few years that improvements in kite structure have been seriously considered, and the recent developments in the art have been largely due to the efforts of one man: "Mr. Laurence Hargrave", of Australia.
Hargrave realized that the structure best adapted for what is called a "good kite" would also be suitable as the basis for the structure of a flying-machine. His researches, published by the Royal Society of New South Wales, have attracted the attention of the world, and form the starting point for modern researches upon the subject in Europe and America.
Anything relating to aerial locomotion has an interest to very many minds, and scientific kite-flying has everywhere been stimulated by Hargrave's experiments.
In America, however, the chief stimulus to scientific kite-flying has been the fact developed by the United States Weather Bureau, that important information could be obtained concerning weather conditions if kites could be constructed capable of lifting meteorological instruments to a great elevation in the free air. Mr. Eddy and others in America have taken the Malay tailless kite as a basis for their experiments, but Professor Marvin, of the United States Weather Bureau; Mr. Rotch, of the Blue Hill Observatory, and many others have adapted Hargrave's box kite for the purpose.
Congress has made appropriations to the Weather Bureau in aid of its kite experiments, and a number of meteorological stations throughout the United States were established a few years ago equipped with the Marvin kite.

Continuous meteorological observations at a great elevation have been made at the Blue Hill Observatory in Massachusetts, and Mr. Rotch has demonstrated the possibility of towing kites at sea by means of steam vessels so as to secure a continuous line of observations all the way across the Atlantic.

HARGRAVE'S BOX KITE

Hargrave introduced what is known as the "cellular construction of kites." He constructed kites composed of many cells, but found no substantial improvement in many cells over two alone; and a kite composed of two rectangular cells separated by a considerable space is now universally known as "the Hargave box kite". This represents, in my opinion, the high-water mark of progress in the nineteenth century; and this form of kite forms the starting point for my own researches (Fig.1).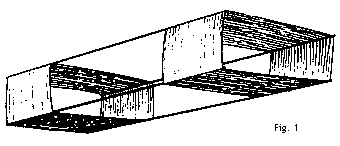
Fig.1

The front and rear cells are connected together by a framework, so that a considerable space is left between them. This space is an essential feature of the kite: upon it depends the fore and aft stability of the kite. The greater the space, the more stable is the equilibrium of the kite in a fore and aft direction, the more it tends to assume a horizontal position in the air, and the less it tends to dive or pitch like a vessel in a rough sea. Pitching motions or oscillations are almost entirely suppressed when the space between the cells is large.
Each cell is provided with vertical sides; and these again seem to be essential elements of the kite contributing to lateral stability. The greater the extent of the vertical sides, the greater is the stability in the lateral direction, and the less tendency has the kite to roll, or move from side to side, or turn over in the air.
In the foregoing drawing I have shown only necessary details of construction, with just sufficient framework to hold the cells together. It is obvious that a kite constructed as shown in Fig.1 is a very flimsy affair. It requires additions to the framework of various sorts to give it sufficient strength to hold the aeroplane surfaces in their proper relative positions and prevent distortion, or bending or twisting of the kite frame under the action of the wind.
Unfortunately the additions required to give rigidity to the framework all detract from the efficiency of the kite:
First, by rendering the kite heavier, so that the ratio of weight to surface is increased; and, secondly, by increasing the head resistance of the kite. The interior bracing advisable in order to preserve the cells from distortion comes in the way of the wind, thus adding to the drift of the kite without contributing to the lift.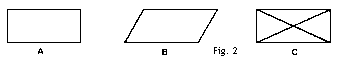
Fig. 2

A rectangular cell like A (Fig.2) is structurally weak, as can readily be demonstrated by the little force required to distort it into the form shown at B. In order to remedy this weakness, internal bracing is advisable of the character shown at C.
This internal bracing, even if made of the finest wire, so as to be insignificant in weight, all comes in the way of the wind, increasing the head resistance without counterbalancing advantages.

TRIANGULAR CELLS IN KITE CONSTRUCTION

In looking back over the line of experiments in my own laboratory, I recognize that the adoption of a triangular cell was a step in advance, constituting indeed one of the milestones of progress, one of the points that stand out clearly against the hazy background of multitudinous details. The following (Fig.3) is a drawing of a typical triangular-celled kite made upon the same general model as the Hargrave box kite shown in Fig.1.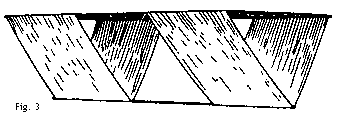
Fig. 3

A triangle is by its very structure perfectly braced in its own plane, and in a triangular-celled kite like that shown in Fig. 3, internal bracing of any character is unnecessary to prevent distortion of a kind analogous to that referred to above in the case of the Hargrave rectangular cell (Fig.2).
The lifting power of such a triangular cell is probably less than that of a rectangular cell, but the enormous gain in structural strength, together with the reduction of head resistance and weight due to the omission of internal bracing, counterbalances any possible deficiency in this respect.
The horizontal surfaces of a kite are those that resist descent under the influence of gravity, and the vertical surfaces prevent it from turning over in the air. Oblique aeroplanes may therefore conveniently be resolved into horizontal and vertical equivalents, that is, into supporting surfaces and steadying surfaces.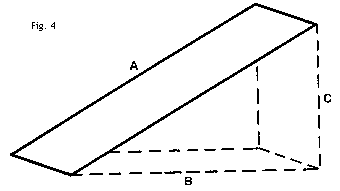

The oblique aeroplane A,for example (Fig.4),may be considered as equivalent in function to the two aeroplanes B and C. The material composing the aeroplane A however, weightless than the material required to form the two aeroplanes B and C, and the framework required to support the aeroplane A weighs less than the two frameworks required to support B and C.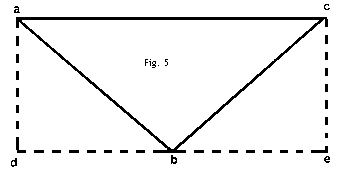

In the triangular cell shown in Fig.5, the oblique surfaces ab, bc, are equivalent in function to the three surfaces ad, de, ec, but weigh less. The oblique surfaces are therefore advantageous.

The only disadvantage in the whole arrangement is that the air has not as free access to the upper aeroplane ac, in the triangular form of cell as in the quadrangular form, so that the aeroplane ac is not as efficient in the former construction as in the latter.
While theoretically the triangular cell is inferior in lifting power to Hargrave's four-sided rectangular cell, practically there is no substantial difference. So far as I can judge from observation in the field, kites constructed on the same general model as the Hargrave Box Kite, but with triangular cells instead of quadrangular, seem to fly as well as the ordinary Hargrave form, and at as high an angle.
Such kites are therefore superior, for they fly substantially as well, while at the same time they are stronger in construction, lighter in weight, and offer less head resistance to the wind.
Triangular cells also are admirably adapted for combination into a compound structure, in which the aeroplane surfaces do not interfere with one another. For example, three triangular-celled kites, tied together at the corners, form a compound cellular kite (Fig.6), which flies perfectly well.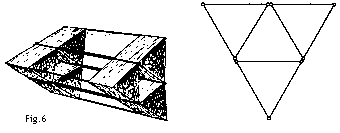
Fig. 6

The weight of the compound kite is the sum of the weights of the three kites of which it is composed, and the total aeroplane surface is the sum of the surfaces of the three kites. The ratio of weight to surface therefore is the same in the larger compound kite as in the smaller constituent kites, considered individually.
It is obvious that in compound kites of this character the doubling of the longitudinal sticks where the corners of adjoining kites come together is an unnecessary feature of the combination, for it is easy to construct the compound kite so that one longitudinal stick shall be substituted for the duplicated sticks.
For example: The compound kites A and B (Fig.7) may be constructed, as shown at C and D, with advantage, for the weight of the compound kite is thus reduced without loss of structural strength. In this case the weight of the compound kite is less than the sum of the weights of the component kites, while the surface remains the same.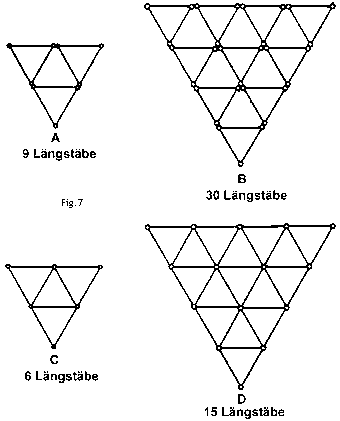
Fig. 7

If kites could only be successfully compounded in this way indefinitely we would have the curious result that the ratio of weight to surface would diminish with each increase in the size of the compound kite. Unfortunately, however, the conditions of stable flight demand a considerable space between the front and rear sets of cells (see Fig.6); and if we increase the diameter of our compound structure without increasing the length of this space we injure the flying qualities of our kite.
But every increase of this space in the fore and aft direction involves a corresponding increase in the length of the empty framework required to span it, thus adding dead load to the kite and increasing the ratio of weight to surface.
While kites with triangular cells are strong in a transverse direction (from side to side), they are structurally weak in the longitudinal direction (fore and aft), for in this direction the kite frames are rectangular.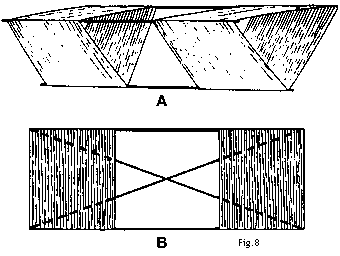

Each side of the kite A, for example (Fig.8), requires diagonal bracing of the character shown at B to prevent distortion under the action of the wind.
The necessary bracing, however, not being in the way of the wind, does not materially affect the head resistance of the kite, and is only disadvantageous by adding dead load, thus increasing the ratio of weight to surface.

THE TETRAHEDRAL CONSTRUCTION OF KITES

Passing over in silence multitudinous experiments in kite construction carried on in my Nova Scotia laboratory, I come to another conspicuous point of advance -- another milestone of progress - the adoption of the triangular construction in every direction (longitudinally as well as transversely); and the clear realization of the fundamental importance of the skeleton of a tetrahedron, especially the regular tetrahedron, as an element of the structure or framework of a kite or flying-machine. Consider the case of an ordinary triangular cell A (Fig.9) whose cross-section is triangular laterally, but quadrangular longitudinally.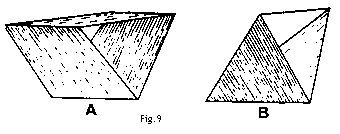
Fig.9

If now we make the longitudinal as well as transverse cross-sections triangular, we arrive at the form of cell shown at B, in which the framework forms the outline of a tetrahedron. In this case the aeroplanes are triangular, and the whole arrangement is strongly suggestive of a pair of birds' wings raised at an angle and connected together tip to tip by a cross-bar (see B, Fig.9; also drawings of winged tetrahedral cells in Fig.10).

A tetrahedron is a form of solid bounded by four triangular surfaces. In the regular tetrahedron the boundaries consist of four equilateral triangles and six equal edges. In the skeleton form the edges alone are represented, and the skeleton of a regular tetrahedron is produced by joining together six equal rods end to end so as to form four equilateral triangles.
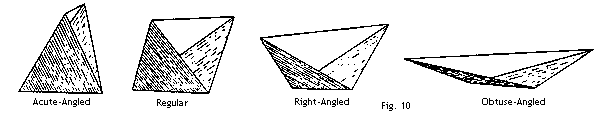Most of us no doubt are familiar with the common puzzle -- how to make four triangles with six matches. Give six matches to a friend and ask him to arrange them so as to form four complete equilateral triangles. The difficulty lies in the unconscious assumption of the experimenter that the four triangles should all be in the same plane. The moment he realizes that they need not be in the same plane the solution of the problem becomes easy. Place three matches on the table so as to form a triangle, and stand the other three up over this like the three legs of a tripod stand. The matches then form the skeleton of a regular tetrahedron(See Fig.11).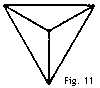
Fig.11

A framework formed upon this model of six equal rods fastened together at the ends constitutes a tetrahedral cell possessing the qualities of strength and lightness in an extraordinary degree. It is not simply braced in two directions in space like a triangle, but in three directions like a solid. If I may coin a word, it possesses " three-dimensional " strength; not " two-dimensional " strength like a triangle, or "one-dimensional" strength like a rod.
It is the skeleton of a solid, not of a surface or a line.

It is astonishing how solid such a framework appears even when composed of very light and fragile material; and compound structures formed by fastening these tetrahedral frames together at the corners so as to form the skeleton of a regular tetrahedron on a larger scale possess equal solidity. Fig.12 shows a structure composed of four frames like Fig.11, and Fig.13 a structure of four frames like Fig.12.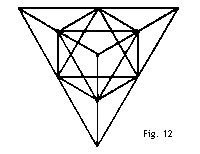

When a tetrahedral frame is provided with aero-surfaces of silk or other material suitably arranged, it becomes a tetrahedra1 kite, or kite having the form of a tetrahedron.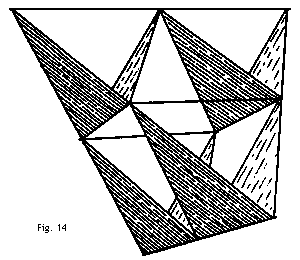

The kite shown in Fig.14 is composed of four winged cells of the regular tetrahedron variety (see Fig. 10), connected together at the corners. Four kites like Fig.14 are combined in Fig.15, and four kites like Fig.15 in Fig.16 (at D).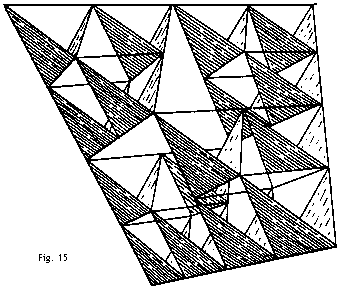

Upon this mode of construction an empty space of octahedral form is left in the middle of the kite, which seems to have the same function as the space between the two cells of the Hargrave box kite. The tetrahedral kites that have the largest central spaces preserve their equilibrium best in the air. The most convenient place for the attachment of the flying cord is the extreme point of the bow. If the cord is attached to points successively further back on the keel, the flying cord makes a greater and greater angle with the horizon, and the kite flies more nearly overhead; but it is not advisable to carry the point of attachment as far back as the middle of the keel. A good place for high flights is a point half-way between the bow and the middle of the keel.
In the tetrahedral kites shown in the plate (Fig.16) the compound structure has itself in each case the form of the regular tetrahedron, and there is no reason why this principle of combination should not be applied indefinitely so as to form still greater combinations.
The weight relatively to the wing-surface remains the same, however large the compound kite may be. The four-celled kite B, for example, weighs four times as much as one cell and has four times as much wing-surface, the 16-celled kite C has sixteen times as much weight and sixteen times as much-wing surface, and the 64-celled kite D has sixty-four times as much weight and sixty-four times as much wing-surface. The ratio of weight to surface, therefore, is the same for the larger kites as for the smaller.
This, at first sight, appears to be somewhat inconsistent with certain mathematical conclusions announced by Prof. Simon Newcomb in an article entitled "Is the Air-ship Coming," published in Mclure's Magazine for September, 1901 -- conclusions which led him to believe that "the construction of an aerial vehicle which could carry even a single man from place to place at pleasure requires the discovery of some new metal or some new force."
The process of reasoning by which Professor Newcomb arrived at this remarkable result is undoubtedly correct.

His conclusion, however, is open to question, because he has drawn a general conclusion from restricted premises.
He says: "Let us make two flying-machines exactly alike, only make one on double the scale of the other in all its dimensions. We all know that the volume, and therefore the weight, of two similar bodies are proportional to the cubes of their dimensions. The cube of two is eight: hence the large machine will have eight times the weight of the other. But surfaces are as the squares of the dimensions. The square of two is four. The heavier machine will therefore expose only four times the wing surface to the air, and so will have a distinct disadvantage in the ratio of efficiency to weight."
Professor Newcomb shows that where two flying-machines - or kites, for that matter - are exactly alike, only differing in the scale of their dimensions, the ratio of weight to supporting surface is greater in the larger than the smaller, increasing with each increase of dimensions. From which he concludes that if we make our structure large enough it will be too heavy to fly.
This is certainly true, so far as it goes and it accounts for my failure to make a giant kite that should lift a man - upon the model of the Hargrave box kite. When the kite was constructed with two cells, each about the size of a small room, it was found that it would take a hurricane to raise it into the air.
The kite proved to be not only incompetent to carry a load equivalent to the weight of a man, but it could not even raise itself in an ordinary breeze in which smaller kites upon the same model flew perfectly well. I have no doubt that other investigators also have fallen into the error of supposing that large structures would necessarily be capable of flight, because exact models of them made upon a smaller scale, have demonstrated their ability to sustain themselves in the air. Professor Newcomb has certainly conferred a benefit upon investigators by so clearly pointing out the fallacious nature of this assumption.
But Professor Newcomb's results are probably only true when restricted to his premises. For models exactly alike, only differing in the scale of their dimensions, his conclusions are undoubtedly sound; but where large kites are formed by the multiplication of smaller kites into a cellular structure the results are very different. My own experiments with compound kites composed of triangular cells connected corner to corner have amply demonstrated the fact that the dimensions of such a kite may be increased to a very considerable extent without materially increasing the ratio of weight to supporting surface; and upon the tetrahedral plan (Fig. 16) the weight relatively to the wing-surface remains the same however large the compound kite may be.
The indefinite expansion of the triangular construction is limited by the fact that dead weight in the form of empty framework is necessary in the central space between the sets of cells (see Fig. 6 ), so that the necessary increase of this space when the dimensions of the compound kite are materially increased - in order to preserve the stability of the kite in the air --adds still more dead weight to the larger structures. Upon the tetrahedral plan illustrated in Figs. 14, 15, 16, no necessity exists for empty frameworks in the central spaces, for the mode of construction gives solidity without it.

Tetrahedral kites combine in a marked degree the qualities of strength, lightness, and steady flight; but further experiments are required before deciding that this form is the best for a kite, or that winged cells without horizontal aeroplanes constitute the best arrangement of aero-surfaces.
The tetrahedral principle enables us to construct out of light materials solid frameworks of almost any desired form, and the resulting structures are admirably adapted for the support of aero-surfaces of any desired kind, size, or shape (aeroplanes or aerocurves, etc. large or small).
Fig.17

In further illustration of the tetrahedral principle as applied to kite construction, I show in Fig.17 a photograph of a kite which is not itself tetrahedral in form, but the framework of which is built up of tetrahedral cells.
This kite, although very different in construction and appearance from the Aerodrome of Professor Langley, which I saw in successful flight over the Potomac a few years ago, has yet a suggestiveness of the Aerodrome about it, and it was indeed Professor Langley's apparatus that led me to the conception of this form.
The wing surfaces consist of horizontal aeroplanes, with oblique steadying surfaces at the extremities. The body of the machine has the form of a boat, and the superstructure forming the support for the aeroplanes extends across the boat on either side at two points near the bow and stern. The aeroplane surfaces form substantially two pairs of wings, arranged dragon-fly fashion. The whole framework of the boat and wings is formed of tetrahedral cells, having the form of the regular tetrahedron, with the exception of the diagonal bracing at the bottom of the superstructure; and the kite turns out to be strong, light, and a steady flyer.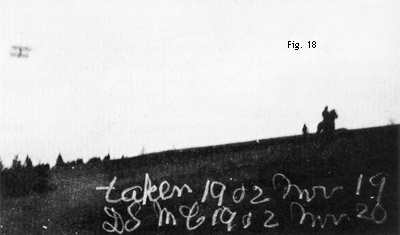
Fig.18

I have flown this kite in a calm by attaching the cord - in this case a Manila rope- to a galloping horse. Fig.18 shows a photograph of the kite just rising in the air. With the horse in the foreground, but the connecting rope does not show. Figure 19 is a photograph of the kite at its point of greatest elevation, but the horse does not appear in the picture. Upon releasing the rope the kite descended so gently that no damage was done to the apparatus lip contact with the ground.
Figure 20 shows a modified form of the same kite, in which, in addition to the central boat, there were two side floats, thus adapting the whole structure to float upon water without upsetting.
An attempt which almost ended disastrously, was made to fly this kite in a good sailing breeze, but a squall struck it before it was let go. The kite went up, lifting the two men who held it off their feet. Of course they let go instantly, and the kite rose steadily in the air until the flying cord (a Manila rope 3/8 inch diameter) made an angle with the horizon of about 45 degree when the rope snapped under the strain.
Tremendous oscillations of a pitching character ensued; but the kite was at such an elevation when the accident happened, that the oscillations had time to die down before the kite reached the ground, when it landed safely upon even keel in an adjoining field and was found to be quite uninjured by its rough experience.

Kites of this type have a much greater lifting power than one would at first sight suppose. The natural assumption is that the winged superstructure alone supports the kite in the air, and that the boat body and floats represent mere dead-load and head resistance.
But this is far from being the case. Boat-shaped bodies having a V-shaped cross-section are themselves capable of flight and expose considerable surface to the wind. I have successfully flown a boat of this kind as a kite without any superstructure whatever, and although it did not fly well, it certainly supported itself in the air, thus demonstrating the fact that the boat surface is an element of support in compound structures like those shown in Fig.17 and 20.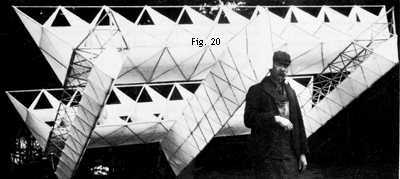
Fig.20

Of course the use of a tetrahedral cell is not limited to the construction of a framework for kites and flying - machines. It is applicable to any kind of structure whatever in which it is desirable to combine the qualities of strength and lightness. Just as we can build houses of all kinds out of bricks, so we can build structures of all sorts out of tetrahedral frames, and the structures can be so formed as to possess the same qualities of strength and lightness which are characteristic of the individual cells. I have already built a house, a framework for a giant wind-break, three or four boats, as well as several forms of kites, out of these elements.
It is not my object in this communication to describe the experiments that have been made in my Nova Scotia laboratory, but simply to bring to your attention the importance of the tetrahedral principle in kite construction.